Subgrade reaction modulus
In foundation design, it is common to assume that the reaction of the soil foundation at each point of the foundation is proportional to its deflection.
The vertical deformation characteristics of the foundation are defined by independent, closely spaced, discrete and linearly elastic springs. This relatively simple mechanical representation of the soil foundation was first introduced by Winkler (1967). The proportionality constant of these springs is known as the subgrade reaction modulus, ks.
The subgrade reaction modulus is a function of the shape and size of the contact surface, the distribution and intensity of the load, and the composition and characteristics of the soil foundation. In the calculation model where the soil is replaced by a spring system (the Winkler model), ks is the constant of proportionality between the additional contact stress q and the displacement w of the point on the surface of the Winkler space:
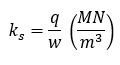
The assumption about the soil as a system of independent springs does not fully correspond to its real behavior. The soil acts as a continuum, wherein the influence from one point is transmitted to the surrounding points. However, the Winkler model has remained in use to this day and is most commonly used in engineering practice.
Using the subgrade reaction modulus
Vertical springs are commonly used in foundation design to calculate the distribution and values of force in a foundation. The value of the subgrade reaction modulus, which represents the long-term differential settlements of the soil foundation is usually used.
Unlike spread footings, strip footings and foundation slab, in the case of, for example, laterally loaded piles, the soil is modeled by a system of horizontal elastic springs.
An appropriate estimation of the subgrade reaction modulus allows for an appropriate estimation of the soil stress distribution under the foundation and, consequently, a more accurate static calculation and dimensioning of the foundation.
The subgrade reaction modulus is not a fundamental property of soil, it depends on:
- the geometry of the loading surface area (loads placed on a larger surface area have an effect on the deeper layers of soil, which can be very compressible).
- the load magnitude.
- the soil stiffness in the impact zone of the load under the foundation.
- the stiffness of the foundation that affects the distribution of pressure
Determining the subgrade reaction modulus
The subgrade reaction modulus can be determined in the following ways:
– using a circular slab
– using tables of characteristic values and correlations
– calculating differential settlements
- Circular slab
Estimating the value using a circular slab for the purpose of calculating the foundation has the disadvantage of only a layer of lesser thickness being loaded onto, compared to a layer loaded with a shallow foundation or a foundation slab. Typically, this is true for the depth of 50 cm, and the impact of the load under the foundation is substantially deeper.
- Correlations
The expression that connects the subgrade reaction modulus with the compressibility modulus Es, which can be determined in the laboratory by examining undisturbed samples, was proposed by Vesić (1961):
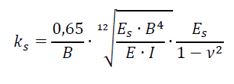
specifically:
Es – soil modulus of elasticity
E – foundation modulus of elasticity
I – foundation moment of inertia
ʋ – Poisson’s ratio for soil
B – width of the foundation
L – length of foundation
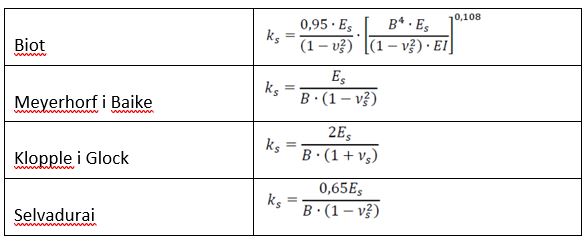
In addition to Vesić, other authors also provided expressions for determining the subgrade reaction modulus:
- Determining the subgrade reaction modulus by differential settlements calculation
The subgrade reaction modulus can be estimated based on the settlement calculation of the actual foundation. The following is an example of a foundation settlement calculation in the Plaxis 3D software package. The settlement of an octagonal reinforced concrete foundation slab (radius of the inscribed circle 20.0 m), 1.50 m thick, loaded with a uniform load of q = 100.0 kN / m2 was calculated.
The soil foundation consists of a soil layer with an elasticity modulus Es = 30.0 MN / m2 and a Poisson’s modulus ν = 0.30.
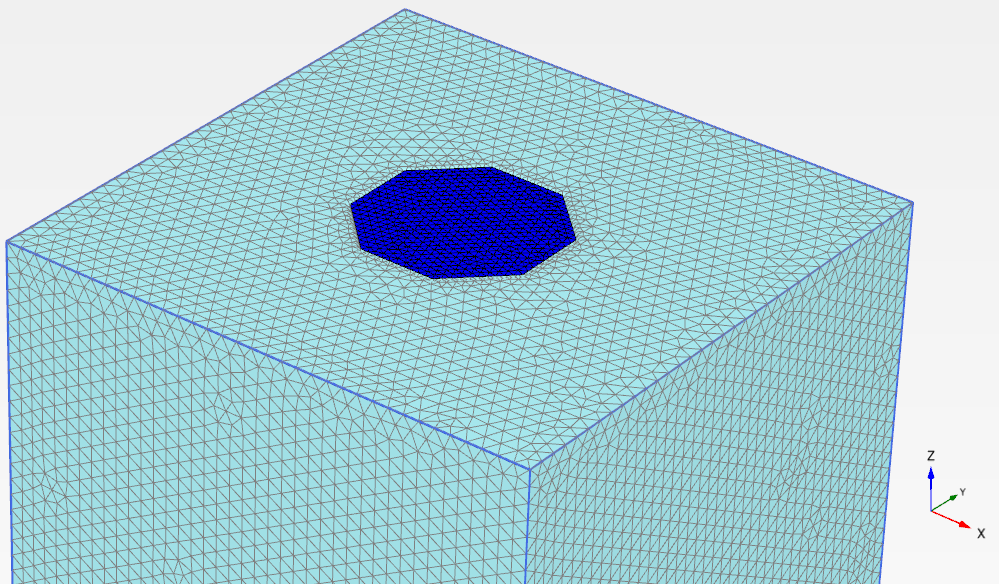
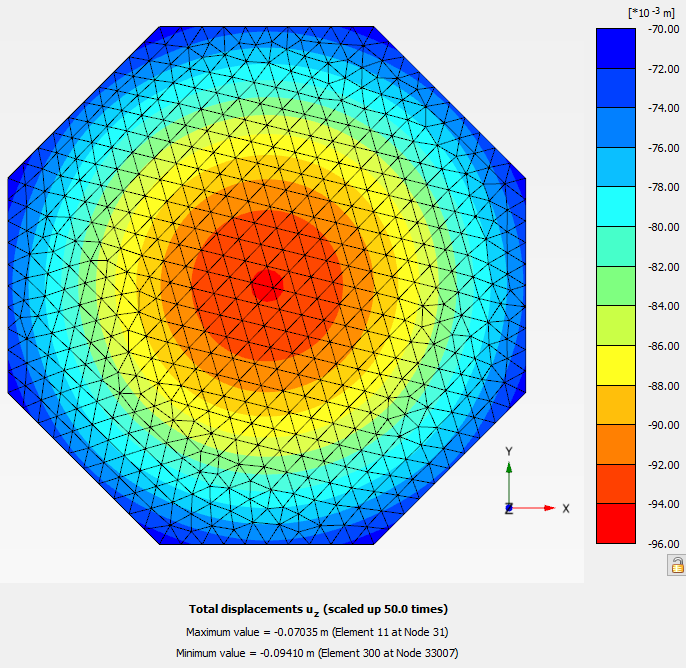
Based on the calculated foundation slab settlement, it can be concluded that the subgrade reaction modulus ks ranges from about 1400.0 kN / m3 at the edge of the slab to about 1060.0 kN / m3 in the middle of the slab. The subgrade reaction model is not a constant value and varies depending on the observed foundation zone.
Conclusion
The subgrade reaction modulus is a function of the shape and size of the contact surface, the distribution and intensity of the load, and the composition and characteristics of the soil foundation. It is the modulus of proportionality between the additional contact stress q and the settlement.
The subgrade reaction modulus can be estimated based on the settlement calculation of the actual foundation. An appropriate estimation of the subgrade reaction modulus allows for an appropriate estimation of the soil stress distribution under the foundation and, consequently, for a more accurate static calculation and dimensional measurement of the foundation.
When choosing the values of the subgrade reaction modulus, engineering experience and good communication between geotechnical and structural engineers is essential.
